Gold has long been prized by humans for its beauty and function, but primarily because of its rarity. And it is not just on earth that gold is rare. Within the universe, it is rarer still, making up only 0.004 parts per billion by atomic count[i]. The reason for this rarity is the extreme conditions required for its production. This report will examine the processes that lead to the formation of gold within the universe, and examine the mechanism behind these processes.
Gold is an element containing 79 protons, and in its only stable isotope, 118 neutrons, for an atomic number (A) of 197 – its atomic structure is shown in Figure 1. Other isotopes of gold have short half-lives[ii], so all discussions of observable gold refer to this stable isotope.
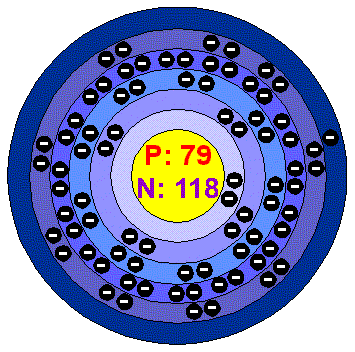
Figure 1. Atomic structure of gold[iii]
Nucleosynthesis
All matter was formed during the first three minutes of the Big Bang, but the extreme conditions means that only hydrogen, helium and trace amounts of lithium were formed, and all other elements have been formed through alternate process – manufactured from these raw materials. This production of elements is termed nucleosynthesis, and it proceeds through a number of different processes – all of them linked to stars[iv]. Within their cores, stars are powered by fusion reactions where lighter nuclei are fused to form heavier nuclei. The mass difference between the resulting products causes the release of energy (via e=mc2) – a so called exothermic reaction. These reactions power the huge energy output of stars. The type of nuclei that can be fused in the star’s core is governed primarily by the temperature of the core, and higher temperatures are required to fuse heavier nuclei. Only stars of sufficient mass are able to generate the gravitational forces that allow heavier nuclei to fuse, and regardless of the mass of the star, this process ceases once iron has been produced in the star’s core. Iron, and more specifically the isotope 56Fe, represents the most stable nuclear configuration, and rather than releasing energy, iron requires the input of energy to fuse with other nuclei, an endothermic process that is non-sustainable. However, as gold, with 79 protons, is much heavier than iron with 26, processes outside stellar core fusion must exist to allow heavier nuclei to be constructed. Two such processes have been identified (several others have been theoretically alluded to[v]), and both were outlined in the ground-breaking work by Burbridge Burbridge Fowler and Hoyle (BBFH) on heavy element nucleosynthesis[vi]. Because of the endothermic nature of 56Fe fusion , and the Coulomb barrier – where positive repulsion is greater than achievable proton energies - heavy element nucleosynthesis is driven by the dual processes of neutron capture and beta-decay.
Key processes in heavy element nucleosynthesis
Neutron capture involves a free neutron being captured by a nucleus. Because neutrons are electrically neutral, they do not have to overcome the Coulomb barrier, and merely require sufficient energy to be incorporated into the nucleus. However, because nuclei are so small (10-15 m across), the neutron has a small target to hit (the size of this target is referred to the neutron capture cross section). To achieve neutron capture, large numbers of neutrons are usually required, the quantity referred to as neutron flux. Beta decay[vii] is the process whereby a neutron within a nucleus is converted to a proton, releasing an electron and an anti-neutrino[viii]. Beta decay is central to heavy element nucleosynthesis, as it increases the proton number (Z) of a nuclei, allowing heavier elements to be constructed. The chart in Figure 2 is central to discussion of nucleosynthesis, and warrants some discussion. It plots the beta decay time frames for various nuclei configurations, with neutron count (N) on the horizontal axis, and proton number (Z) on the vertical. The beta decay time frame is a measure of a nuclei’s stability. The thin black line running through the chart is referred to as the valley of stability, and describes the proton/neutron configurations that form the most stable nuclear isotopes A N/Z combination either side of this line will decay towards it.
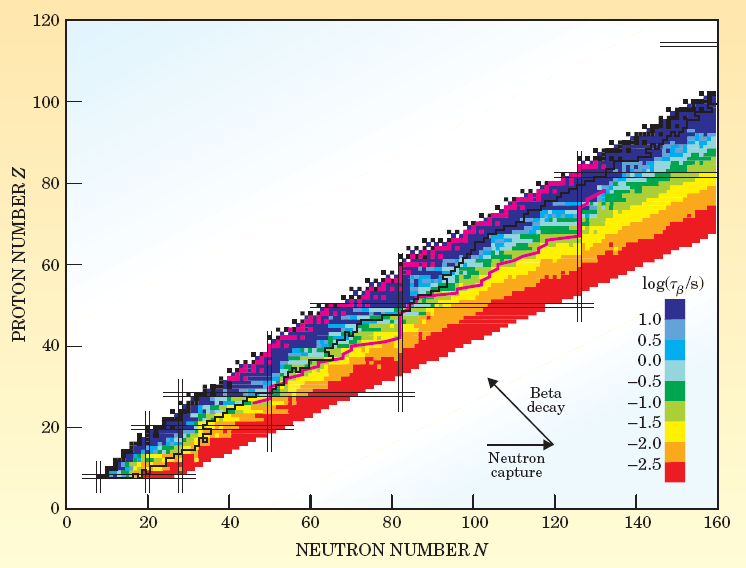
Figure 2. Isotope stability of nuclei configurations[ix]
We can see that neutron capture will move a nuclei horizontally along the axis, and the further it moves horizontally from the valley of stability, the shorter the time scale before beta decay occurs. Beta decay, by increasing the proton number and reducing the neutron number, will shift the nucleus up and to the left, creating a different nuclei with the same atomic number. Finally, the right hand edge of the plotted area is referred to as the neutron drip feed line – the point at which nuclei will lose neutrons as quickly as they are gained – the theoretical saturation point for neutron capture. BBFH identified two processes that would lead to heavier element synthesis via neutron capture – the s-process and the r-process, referring to ‘slow’ and ‘rapid’ respectively. The key determinant of which process occurs is the relationship between time between neutron captures (Tn) and time between beta decay events (Tb). Tn is dependant on neutron flux, whereas Tb is dependant only on nuclear species. Predictions of s-process contribution to gold nucleosynthesis are about 5.8%, so our discussions of s-process will be limited.
s-process nucleosynthesis
The s-process is characterized by neutron captures taking place slowly, allowing beta decay to occur between captures. Indeed, models of the s-process typical ascribe 1000’s of years between capture events.[x] This means that nuclei slowly move up the proton count chain, with successive capture and decay events. The process stays very close to the valley of stability. Single neutron captures by a nuclei can be readily reproduced in the laboratory, and this process is well studied and verified. The s-process is believed to take place in late-stage intermediate mass stars (0.8 – 8 solar masses), although new evidence is emerging that Asymptotic Giant Branch stars may also be production sites, based on analysis of the stellar atmospheres of companion stars[xi].

Figure 3. Elemental Abundances from s- and r-processes[xii]
r-process nucleosynthesis
Unlike that s-process, the r-process cannot be simulated in laboratories, and its operations are not fully understood. However, there is a large body of evidence that points to Type II supernova as the chief site of r-process nucleosynthesis in the universe, with neutron star collision proposed as a lesser contributing mechanism. The primary reason for suspecting Type II supernova is the high neutron flux required, which would require an explosive event. Our understanding of r-process reactions during supernova are limited on several fronts – particularly the difficulty of accurately modeling supernova, and the lack of experimental data on nuclei decay rates of the very unstable nuclei that are produced as neutron capture approaches the neutron drip feed line. r-process nucleosynthesis must occur in conditions where sufficient neutron flux exists so that additional neutron captures can occur before beta decay commences, meaning that more unstable nuclei with greater numbers of neutron can be created – approaching the neutron drip line. Events that generate sufficient neutron flux to allow this level of capture are not sustainable of long-periods, and once the neutron flux drops, beta decay can proceed, allowing the neutron laden nuclei to move back towards the valley of stability via beta decay. However, the higher number of neutrons involved in the beta decay process means that much higher Z numbers can be achieved in much shorter timeframes (essentially the duration of the neutron flux event plus the duration of the subsequent beta decays), and much higher Z numbers can be achieved more readily (heavier elements from Polonium to Uranium[xiii] are produced exclusively through the r-process). Gold, with 79 protons is one such element that is far more readily produced through r-process nucleosynthesis than s-process, and approximately 95% of gold is produced via this mechanism[xiv]. No definitive decay path for gold has been identified, and the limited scope of supernova modeling does not allow this. Simply stated, providing the equations are balanced, then any neutron capture and decay process that leaves 79 protons and 118 neutrons can be a candidate, such as the beta-decay of 197Hg.
Supernova induced r-process nucleosynthesis
The collapse of a massive star (>8 solar masses) triggers several processes that are important for creating conditions where the r-process can occur. The massive gravitational collapse of the iron core as the fusion products are exhausted overcomes the electron degeneracy pressure, causing the electrons and protons in the core to merge into neutrons, releasing a neutrino. The large volumes of atoms converted trigger massive neutrino emissions – 1057 in the case of SN 1987 A[xv]. Quantum stiffening in the newly created neutron core creates a shockwave that propagates out from the core, striking the outer layers of the dying star. Finally, gravitational collapse releases huge amounts of energy at very high temperatures, which through gamma ray reactions, produce an even greater quantity of neutrinos (1058 in SN 1987 A[xvi]), which push out from the core, and encounter the slowing shockwave and the matter in front of it.
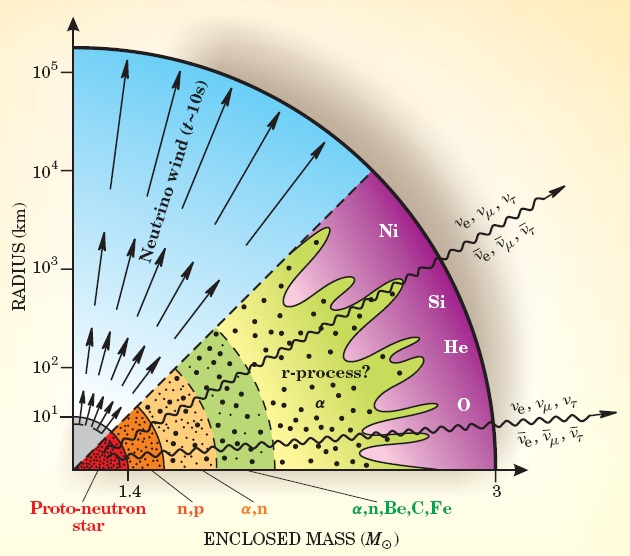
Figure 4. r-process nucleosynthesis in Type II supernova[xvii]
The energy provided from the neutrino flux interacting with the high density matter at the front of the shockwave (the shockwave compresses the material into ultrahigh densities, increasing the likelihood of the otherwise unlikely neutrino interaction) triggers beta-decay, both plus and minus, creating a sea of protons and neutrons as end-products that create the source neutrons for r-process capture. The heavier r-process elements are synthesized at a temperature of about 109 K and an assumed neutron density of 1020-1030 per cm3[xviii]. During supernova r-process formation, high neutron flux are accompanied by large quantities of gamma rays, causing a process called photodisintegration to take place – the neutrons are literally stripped from the nuclei. In supernovae environments, both neutron capture and photodisintegration take place in time scales shorter than those for beta decay, meaning that the interplay between these two process determines the rate of neutron capture – and therefore the maximum abundance along the isotope chain (the distribution of isotopes – being determined by the number of neutrons in the nucleus), and the nuclear configurations that remain when the neutron flux disappears – as it must do for such brief explosive events. Because of the large number of beta-decay events required for 56Fe to form 197Au, a single capture/decay sequence is not feasible. Instead, the duration of a supernova driven high-neutron flux will exceed the beta decay times for a number of the unstable nuclei produced, and once again visiting Figure 2, we see that decay times decrease as the neutron drip line is approach – therefore, the more neutrons captured, the more likely the occurrence of beta-decay. The product of beta-decay in this time frame may not necessarily be stable (in fact it is unlikely to be), but will be more readily able to capture neutrons in a continuous cycle of capture and decay. Thus, gold would not be formed from a massive beta-decay from a neutron abundant iron nuclei, but beta decay within a much more proton rich nuclei, such as 198Hg, which itself would have been the end-result of a long sequence of capture and decay events. Added to the neutron capture/beta decay process is the operation of fission on heavier elements, causing heavier elements to break into lighter elements “when neutron –rich nuclei are produced at excitation levels above their fission barriers.”[xix] The fission products may themselves be recycled into seed nuclei for further capture/decay, or may contribute to the final abundances of an r-process event. The excitation energy determines the heaviest nuclei that can be produced in an r-process event. The r-process is terminated by neutron-induced fission[xx], and its presence is a further complicating factor in r-process modeling. The presence of heavier elements in the spectroscopes of halo stars indicates that r-process must have contributed these elements, as the lifetimes of these stars preclude the contribution of significant amounts of heavier elements through s-process mechanism.
Neutron star collisions
Studies[xxi] have indicated that providing neutron:proton ratios are sufficiently high, within a system having appropriately high entropy, r-process mechanisms can be sustained. The merger of a neutron star (an extremely good source of neutron) with a companion star (another neutron star, a black hole, or possibly a white dwarf), would compensate for lower entropy through very high neutron levels – densities such as 1033 per cm3, which could allow r-process chains resulting in heavy element production. Incomplete studies in this area indicate no nuclei lighter than A=130 would be produced. Coupled with the rarity of such an occurrence, the contribution of this process to nucleosynthesis of gold and other heavy elements is limited.
Conclusion
Due to the large number of protons in its nucleus, gold relies on very convoluted, rare and violent processes to be produced – predominantly the r-process occurring in Type II supernova. The huge energy releases that create gold also drive its distribution in the galaxy, allowing it to mix into proto-star masses, and in the case of the solar system, contribute to the formation of planets, where it was available for humans to discover and covet.
[i] http://www.webelements.com/webelements/elements/text/Au/geol.html [ii] The longest half life for an unstable isotope is 186 days for Au195 [iii] http://www.chemicalelements.com/elements/au.html [iv] Gamma ray collisions can produce Beryllium and Boron [v] The p-process is one example, where high proton densities and temperatures cause nuclei to move toward the proton drip feed line and return to the valley of stability via beta+ decay. [vi] “Reviews of Modern Physics”, October 1957 [vii] This example refers to beta-minus decay. Beta-plus decay, where a proton is converted into a neutron and a positron (plus an anti-neutrino), is also viable, although only within certain nuclei. [viii] This process is driven by the weak force, and involves a down quark being converted to a up quark through the emission of a W-boson [ix] http://www.nhn.ou.edu/~cowan/pt_Cowan10_2004.pdf [x] http://www.absoluteastronomy.com/ref/s-process [xi] http://www-astro.ulb.ac.be/~siess/papers/pasa2003.pdf [xii] http://www.nhn.ou.edu/~cowan/pt_Cowan10_2004.pdf [xiii] http://www.ucolick.org/~bolte/AY4/notes12/node1.html [xiv] Lead is an exception here, as its nuclear configuration has full neutron and proton layers, which give long decay times, and therefore concentrate nuclei production in this configuration, allowing larger contributions from the s-process [xv] Gribbin, J., “stardust”, Yale University Press, 2000, p 173 [xvi] Gribbin, p174 [xvii] http://www.nhn.ou.edu/~cowan/pt_Cowan10_2004.pdf [xviii] http://www.site.uottawa.ca:4321/astronomy/index.html#nucleosyntheticreaction [xix] http://www.nhn.ou.edu/~cowan/pt_Cowan10_2004.pdf [xx] currently believed to be in the region of A = 270 - http://en.wikipedia.org/wiki/R-process [xxi] http://www.nhn.ou.edu/~cowan/pt_Cowan10_2004.pdf

